Equal Area Criterion

The equal area criterion is a simple graphical method for concluding the transient stability of two-machine systems or a single machine against an infinite bus. This principle does not require the swing equation for the determination of stability conditions. The stability conditions are recognized by equating the areas of segments on the power angle diagram between the p-curve and the new power transfer line of the given curve.

The principle of this method consists on the basis that when δ oscillates around the equilibrium point with constant amplitude, transient stability will be maintained.

Starting with swing equation

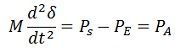where, M = Angular Momentum
P_E = Electrical Power
P_S = Mechanical Power
δ= Load Angle

Multiplying both sides of the above equation by dδ/dt, we get

                                                                  or

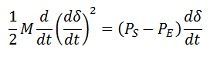Rearranging, multiplying by dt and integrating, we have

Where δ_o, is the torque angle at which the machine is operating while running at synchronous speed under normal conditions. Under the above conditions, the torque angle was not changing i.e. before the disturbance. 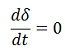 Also, if the system has transient stability the machine will again operate at synchronous speed after the disturbances, i.e.,

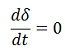Hence the condition for the transient state stability is given by the equation

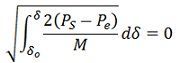
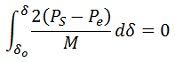
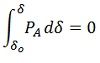
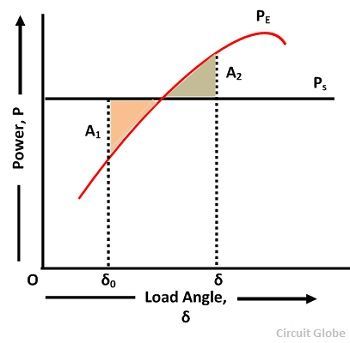The area A₁ represents the kinetic energy stored by the rotor during acceleration, and the A₂ represents the kinetic energy given up by the rotor to the system, and when it is all given up, the machine has returned to its original speed.

The area under the curve P_A should be zero, which is possible only when P_A has both accelerating and decelerating powers, i.e., for a part of the curve P_S> P_E and for the other P_E> P_S. For a generation action, P_S> P_E for the positive area and  A₁> P_S for negative areas A₂ for stable operation. Hence the name equal area criterion.

The equal area criterion is also used for determining the maximum limit on the load that the system can take without exceeding the stability limit. This can happen only when the area between the P_S line and the P_E curve is equal to the area between the P_S line, and the P_E curve is equal to the area between the initial torque angle δ₀ and the line P_S. In this case, the area A₂ is less than the area A₁; the system will become unstable.

The problems associated with the transient stability of the system is because of the following reasons. These are as follows

• Sudden change in load
• Switching off one of the lines which cause a change in the reactance of the system and hence a change in load conditions.
• Sudden fault on the system which causes the reduction in output, requiring an arrangement for clearance for the clearance of the fault rapidly, and study of after fault condition which may cause part of the system outage.

In each case, the procedure will be to determine the power angle curve for the initial conditions of the system, for the conditions under fault, and for the after fault condition and plot the curve in per unit value. Then locate the points for the load initial conditions finding out δ_o. Then, using equal area criterion, determine the new angle of displacement δ. The maximum angle δ_max which may be allowed and the corresponding maximum permissible load can also be determined