Circle Diagram of an Induction Motor

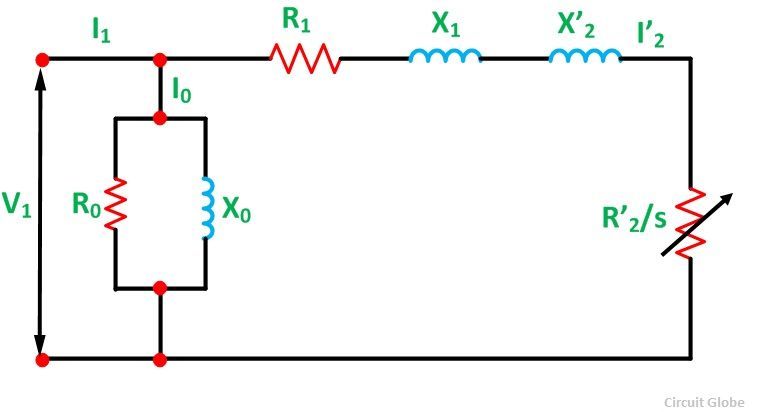
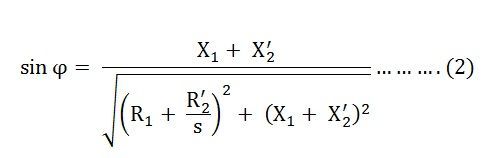The Circle Diagram of an Induction motor is very useful to study its performance under all operating conditions. The construction of the circle diagram is based on the approximate equivalent circuit shown below. It is the diagrammatic representation of the performance of the induction motor. The circle diagram provides information about the power output, losses, and the efficiency of the induction motor.

Contents:

• Construction of the Circle Diagram
• Result Obtained from the Circle Diagram
• Significance of lines on the Circle Diagram

Applying KCL (Kirchhoff’s Current Law)

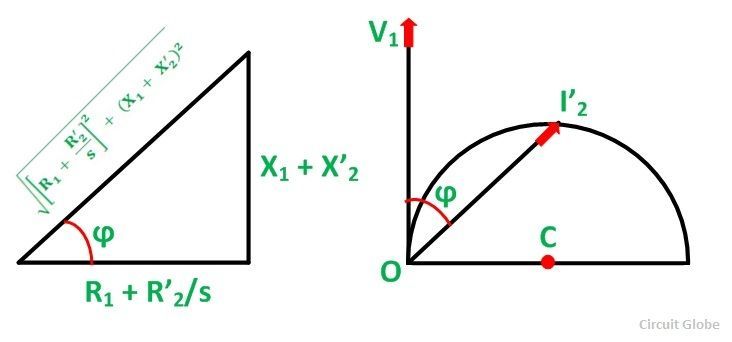
Let the phase voltage V₁ be taken along the vertical axis OY as shown in the figure below:

The No-Load current I₀ = OA lags behind V₁ by an angle ϕ₀. The no-load power factor angle ϕ₀ is of the order of 60 to 80 degrees because of the large magnetizing current needed to produce the required flux pole in a magnetic circuit containing the air gaps.

At the no-load condition, s = 0 and R₂/s are infinite, or we can say that the R₂/s is an open circuit at no load.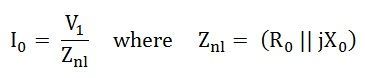

Here, all the rotational losses are considered under R₀ and the no-load loss is given by the equation shown below:

The rotor current referred to the stator is given by: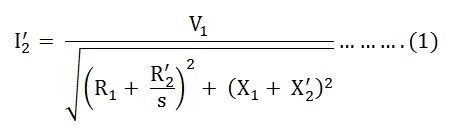

The current I’₂ lags behind the voltage V₁ by the impedance angle ϕ as shown in the figure below:

Where,

Combining equation (1) and (2) we get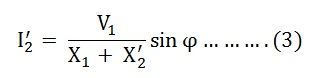

The above equation (3) is of the form r = a sin φ which represents a circle in polar form with the diameter a.

From the above figure (b) shown, the following points are illustrated.

• The locus of I’₂ is a circle of the diameter V₁/ X₁ + X’₂
• The radius of the circle O’C = V₁/ 2(X₁ + X’₂)
• The center C has the coordinates [V₁/ 2(X₁ + X’₂), 0]

The resulting circle diagram of the induction motor is shown below:

It is seen that the tip of the phasor I₁ coincides with that of the phasor I’₂. Thus, the locus of both I₁ and I’₂ is the upper semicircle. I₁ and I’₂ radiate from the origin O and O’ respectively. When the motor is started s = 1 with the rated voltage, the tip of I₁ and I’₂ will be at some point F of the circle.

As the motor accelerates, the tips of I₁ and I’₂ move around the circle in an anti-clockwise direction. This process continues until the output torque matches the load torque. If there is no shaft load, the motor accelerates to synchronous speed. At this point I’₂ = 0 and I₁ = 0.

Construction of the Circle Diagram

The following data are required for constructing the circle diagram:

• Stator phase voltage V₁ = V_L/√3
• No-load current I₀
• No-load power factor cosϕ₀
• Blocked rotor current and power factor
• Stator phase resistance R₁.

Steps to draw Circle Diagram of an Induction Motor:

• Take the phasor voltage V₁ along the y-axis.
• Choose a convenient current. With O as origin, draw a line OO’ = I₀ at an angle ϕ₀ with V₁.
• Draw the line OKN perpendicular to V₁. Similarly, draw a line O’D perpendicular to V₁.
• From point O draw the line equal to the blocked rotor current I_1BR to the same scale as I₀. This line lags behind V₁ by the blocked rotor power factor angle ϕ_1BR.
• Join O’F and measure its magnitude in amperes. The line O’F represents I’_2BR.
• From point F, draw a line FMN parallel to V₁. This line is perpendicular to O’D and ON.
• Calculate MS = I’²_2BRR₁/V₁ and locate point S. Join O’S and extends it to meet the circle at J.
• Draw the perpendicular bisector of the chord O’F. This bisector will pass through the center of the circle at point C. Now with the radius CD’ or CD draw the circle.

Result Obtained from the Circle Diagram

Assume that the line current I₁ is known. With the centre at O, draw an arc with the radius I₁. This arc intersects the circle at the operating point E. Draw the line EK and locates the point H, L, G.

The following results are obtained from the circle diagram shown above.

• Input power = 3V₁ KE
• Rotational losses = 3V₁ KH
• Stator copper loss = 3V₁ HL
• Rotor copper loss = 3V₁ LG
• Output power = 3V₁ GE
• Output torque = 3V₁ LE/ω_s
• Starting torque = 3V₁ SF/ω_s
• Slip = LG/LE
• Speed = GE/LE X n_s
• Efficiency = GE/KE
• Power factor = KE/OE

Significance of lines on the Circle Diagram

Input line ON

The vertical distance between any point on the circle and the line ON represents the input power. Therefore, line ON is called the input line.

Output Line O’F

The vertical distance between any point on the circle and the line O’G represents the output power. Hence, line O’F is called the output line.

Air Gap Power Line O’J

Line EL represents the air gap power P_g; line O’L is referred to as the air gap power line. Since, Ʈ_d = P_g/ ω_s. This line is also known as Torque line.