Two Reaction Theory – Salient Pole Synchronous Machine

Two Reaction Theory was proposed by Andre Blondel. The theory proposes to resolve the given armature MMFs into two mutually perpendicular components, with one located along the axis of the rotor of the salient pole. It is known as the direct axis or d axis component. The other component is located perpendicular to the axis of the rotor salient pole. It is known as the quadrature axis or q axis component.

The d axis component of the armature MMF, F_a is denoted by F_d, and the q axis component by F_q. The component F_d is either magnetizing or demagnetizing. The component F_q results in a cross-magnetizing effect. If Ψ is the angle between the armature current I_a and the excitation voltage E_f and F_a is the amplitude of the armature MMF, then

Salient Pole Synchronous Machine Two Rection Theory

In the cylindrical rotor synchronous machine, the air gap is uniform. The pole structure of the rotor of a salient pole machine makes the air gap highly non-uniform. Consider a 2 pole, salient pole rotor rotating in the anticlockwise direction within a 2 pole stator as shown in the figure below:

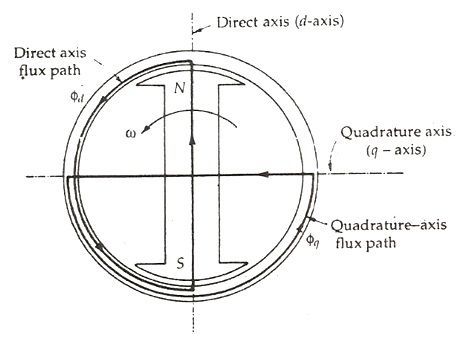The axis along the axis of the rotor is called the direct or the d axis. The axis perpendicular to the d axis is known as the quadrature or q axis. The direct axis flux path involves two small air gaps and is the path of the minimum reluctance. The path shown in the above figure by ϕ_q has two large air gaps and is the path of the maximum reluctance.

The rotor flux B_R is shown vertically upwards as shown in the figure below:

The rotor flux induces a voltage E_f in the stator. The stator armature current I_a will flow through the synchronous motor when a lagging power factor load is connected to it. This stator armature current I_a lags behind the generated voltage E_f by an angle Ψ.

The armature current produces stator magnetomotive force F_s. This MMF lags behind I_a by angle 90 degrees. The MMF F_S produces stator magnetic field B_S long the direction of Fs. The stator MMF is resolved into two components, namely the direct axis component F_d and the quadrature axis component F_q.

If,

• ϕ_d is the direct axis flux
• Φ_q is the quadrature axis flux
• R_d is the reluctance of the direct axis flux path

Therefore

As, R_d < R_q, the direct axis component of MMF F_d produces more flux than the quadrature axis component of the MMF. The fluxes of the direct and quadrature axis produce a voltage in the windings of the stator by armature reaction.

Let,

• E_ad be the direct axis component of the armature reaction voltage.
• E_aq be the quadrature axis component of the armature reaction voltage.

Since each armature reaction voltage is directly proportional to its stator current and lags behind by 90 degrees angles. Therefore, armature reaction voltages can be written as shown below:

Where,

• X_ad is the armature reaction reactance in the direct axis per phase.
• X_aq is the armature reaction reactance in the quadrature axis per phase.

The value of X_ad is always greater than X_aq. As the EMF induced by a given MMF acting on the direct axis is smaller than for the quadrature axis due to its higher reluctance.

The total voltage induced in the stator is the sum of EMF induced by the field excitation. The equations are written as follows:

The voltage E’ is equal to the sum of the terminal voltage V and the voltage drops in the resistance and leakage reactance of the armature. The equation is written as: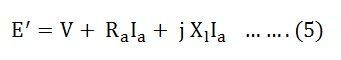

The armature current is divided into two components; one is the phase with the excitation voltage E_f and the other is in phase quadrature to it.

If

• I_q is the axis component of I_a in phase with E_f.
• I_d is the d axis I_a lagging E_f by 90 degrees.

Therefore,

Combining the equation (4) and (5) we get,

Combining the equation (6) and (7) we get, Let,

The reactance X_d is called the direct axis synchronous reactance, and the reactance X_q is called the quadrature axis synchronous reactance.

Combining the equations (9) (10) and (11), we get the equations shown below: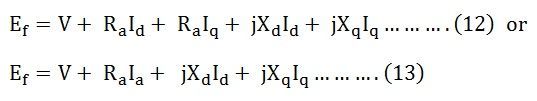 The equation (12) shown above is the final voltage equation for a salient pole synchronous generator.