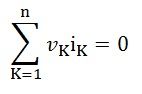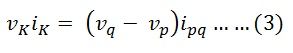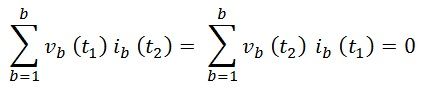Tellegen’s Theorem

Tellegen’s Theorem states that the summation of power delivered is zero for each branch of any electrical network at any instant of time. It is mainly applicable for designing the filters in signal processing.

It is also used in complex operating systems for regulating stability. It is mostly used in the chemical and biological system and for finding the dynamic behaviour of the physical network.

Contents:

• Explanation of Tellegen’s Theorem
• Steps for Solving Networks Using Tellegen’s Theorem
• Application of Tellegen’s Theorem

Tellegen’s theorem is independent of the network elements. Thus, it is applicable for any lump system that has linear, active, passive and time-variant elements. Also, the theorem is convenient for the network which follows Kirchoff’s current law and Kirchoff’s voltage law.

Explanation of Tellegen’s Theorem

Tellegen’s Theorem can also be stated in another word as, in any linear, nonlinear, passive, active, time-variant or time-invariant network the summation of power (instantaneous or complex power of sources) is zero.

Thus, for the K^th branch, this theorem states that:

Where,
n is the number of branches
v_K is the voltage in the branch

i_K is the current flowing through the branch.

Let,

Equation (1) shows the Kth branch through current

v_K is the voltage drop in branch K and is given as:

Where v_p and v_q are the respective node voltage at p and q nodes.
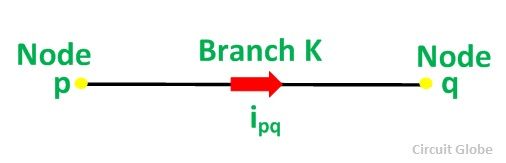We have,

Also,

Obviously

Summing the above two equations (2) and (3), we get

Such equations can be written for every branch of the network.

Assuming n branches, the equation will be:

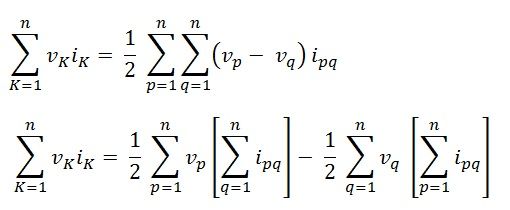
However, according to the Kirchhoff’s current law (KCL), the algebraic sum of currents at each node is equal to zero.

Therefore,

Thus, from the above equation (4) finally, we obtain

Thus, it has been observed that the sum of power delivered to a closed network is zero. This proves the Tellegen’s theorem and also proves the conservation of power in any electrical network.

It is also evident that the sum of power delivered to the network by an independent source is equal to the sum of power absorbed by all passive elements of the network.

Steps for Solving Networks Using Tellegen’s Theorem

The following steps are given below to solve any electrical network by Tellegen’s theorem:

• Step 1 – In order to justify this theorem in an electrical network, the first step is to find the branch voltage drops.
• Step 2 – Find the corresponding branch currents using conventional analysis methods.
• Step 3 – Tellegen’s theorem can then be justified by summing the products of all branch voltages and currents.

For example, if a network having some branches “b” then:

Now, if the set of voltages and currents is taken, corresponding the two different instants of time, t₁ and t₂, then Tellegen’s theorem is also applicable where we get the equation as shown below:

Application of Tellegen’s Theorem

The various applications of the Tellegen’s theorem are as follows:

• It is used in the digital signal processing system for designing filters.
• In the area of the biological and chemical process.
• In topology and structure of reaction network analysis.
• The theorem is used in chemical plants and oil industries to determine the stability of any complex systems.

This is all about Tellegen’s theorem.